Pythagoras hypothized that the same mathematical relations present in music scales and harmony were also present in celestial bodies, and thus in nature. He called this concept "The Music of Spheres".
To study those relations, he used a monochord. This musical instrument became the first widely known harmonic oscillator in Western science.
In ancient China, it was through bells and pipes that they studied how sound revealed derived and consistent mathematical fractions.
Similar to what happened in the West, Chinese divided the octave in 12 pitches, as they did with year in 12 lunar months, and the day in 12 fractions of double hours.
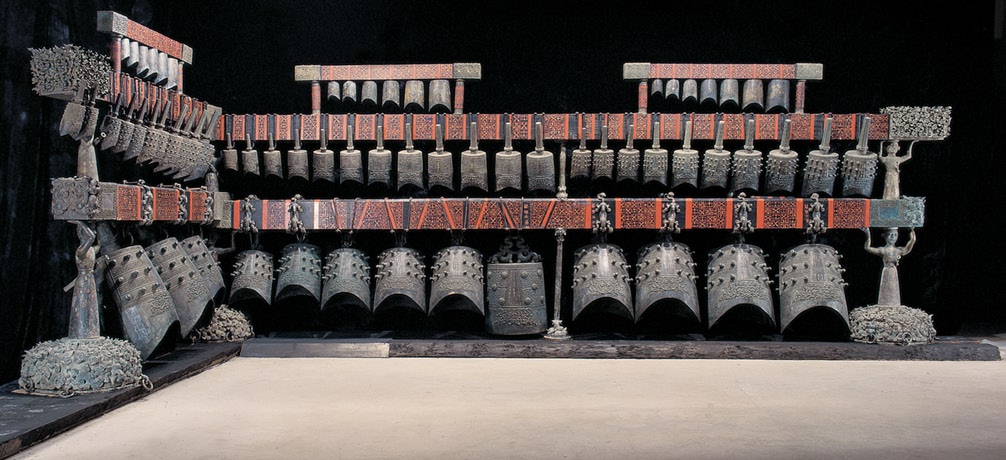Bianzhong of Marquis Yi of Zeng (曾侯乙编钟) ancient musical instrument of 65 bronze bells dating from 433 B.C. It shows a 12 tone scale system.
A touchstone of classical mechanics, Newton's second law of motion (F=ma) formulated with the help of pendulums was fundamental for understanding simple harmonic motion. Not suprisingly Newton also explored tuning systems in order to solve the question of the so-called Pythagorean comma (a disadjustment that happens along the piling up of the musical interval of the 5th, which eventually do not fit into perfect octaves) which puzzled scholars since ancient China.
In the 19th century, studies of harmony and resonance through harmonic oscillator models were pivotal in the development of Maxwell's revolutionary theory of electromagnetism, which unified light, electricity, and magnetism. Maxwell's theories, in turn, paved the way for Planck's quantum theory by advancing the study of electromagnetic emissions from blackbodies, ultimately laying the groundwork for quantum mechanics.
In the 20th Century, Schröedinger's harmonic oscillator proved fundamental to study subatomic particles. Those particles draw 3D patterns similar to Chladni figures, and are described as wavefunctions containing the wave probable energy and position.
(Modified p5 script from original version by JPonce)
Chladni patterns had an impact on the development of wave theories (and to electromagnetism and quantum physics) by visualizing sound and hence demostrate that waves are no abstract phenomena, but material manifestations.
(Modified p5 script from original version by fepegar)
The Harmonograph draw Lissajous figures which are formed by combining two perpendicular harmonic oscillations. These curves became essential in understanding signal analysis and wave interference and offered an early glimpse into nonlinear dynamics, in which patterns vary depending on initial conditions and damping factors. This inspired later work in chaos theory, where systems can exhibit orderly yet sensitive dependence on initial conditions. Van der Pol, in the 1950s, created a non-linear oscillator where the restoring force is not directly proportional to the displacement. This oscillator more suitable for biological systems such as the rhythms of the heart and neural oscillations in the brain.